|
Maths Class X
1997(CBSE)
You are on Set no 1 Qno. 1 to 20
Time allowed : 3 hours
Maximum Marks :
100
General Instructions :
(i) Question number 1 to 15 carry
2 marks each.
(ii) Question number 16 to 25 carry 4 marks
each.
(iii) Question number 26 to 30 carry 6 marks each.
(iv) Write
the serial number of the question before attempting it.
(v) Use of
logarithmic and trignometric tables is permitted. Use of calculator is not
permitted.
Section - A
Q1) Determine the value of
 for which
the following system of linear equations has an infinite number of
solutions :
x + 3y = - 3; 12x + y = (Marks 2)
Q2)
Find the g.c.d. of the
following polynomials :
p(x) = 8(x4 - 16); q(x) =
12(x3 - 8) (Marks 2)
Q3)
If b is the mean
proportional between a and c, prove that
(a2 - b2
+ c2)/(a-2 - b-2 + c-2) =
b4 (Marks 2)
Q4)
Find the values of k so
that the quadratic equation
x2 - 2x(1 - 3k) + 7(3 + 2k) =
0
has equal roots. (Marks 2)
Q5)
OABC is a rhombus whose
three vertices A, B, and C lie on a circle with centre O. If the radius of
the circle is 10 cm, find the area of the rhombus. (Marks
2)
Q6)
Lata goes to a shop to
buy a leather coat, costing Rs. 654. The rate of sales tax is 9%. She
tells the shopkeeper to reduce the price of the coat to such an extent
that she has to pay Rs. 654, inclusive of sales tax. Find the reduction
needed in the price of the coat. (Marks 2)
Q7)
If cos  + sin =  2cos , show that
cos - sin = 2sin . (Marks 2)
Q8)
Show that
(1 +
(1/tan2 ))(1 + (1/cot2 ) = 1/(sin2 - sin4 ) (Marks
2)
Q9)
In Fig. 1, ABCD is a
rectangle with AD = 12 cm and DC = 20 cm. Line segment DE is drawn making
an angle of 30o with AD, intersecting AB in E. Find the lengths
of DE and AE. (Marks 2)
Q10)
If the diagonal BD of a
quadrilateral ABCD bisects both  B and D, show that
AB/BC = AD/CD. (Marks 2)
Q11)
In Fig. 2, ABC is a
triangle in which BAC = 30o. Show that BC is the radius of the
circum-circle of  ABC, whose centre is O. (Marks 2)
Q12)
In two similar triangles
ABC and PQR, if their corresponding altitudes AD and PS are in the ratio
of 4 : 9, find the ratio of the area of ABC to that of PQR. (Marks
2)
Q13) If the mean of n
observations x1, x2, x3, ...,
xn is 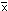 , prove that the mean of the observations x1 + a,
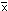x2 + a, x3 + a, ..., xn + a is + a. (Marks
2)
Q14)
Flow Chart. Omitted
being out of Syllabus. (Marks 2)
Q15)
Fill in the blanks in
the following table and find the Crude Death Rate (CDR) for the
data: (Marks 2)
Age
group
(in years) |
Population |
Number
of
Deaths |
| 0 -
15 |
4,000 |
200 |
| 15 -
25 |
- |
120 |
| 25 -
40 |
1,500 |
100 |
| 40 -
50 |
3,000 |
150 |
| Above
50 |
2,000 |
- |
| Total |
13,000 |
700 |
Section - B
Q16) Solve graphically the
following system of linear equations:
x + y = 3, 2x + 5y = 12
(Marks 4)
Q17)
Factorise. Omitted,
being out of Syllabus. (Marks 4)
Q18) A part of monthly
expenses of a family is constant and the remaining varies with the price
of wheat. When the rate of wheat is Rs. 250 a quintal, the total monthly
expenses of the family are Rs. 1,000 and when it is Rs. 240 a quintal, the
total monthly expenses of the family are Rs. 980. Find the total monthly
expenses of the family when the cost of wheat is Rs. 350 a quintal.
(Marks 4)
Q19)
A piece of cloth costs
Rs. 200. If the piece were 5 m longer, and each metre of cloth costed Rs.
2 less, the cost of the piece would have remained unchanged. How long is
the piece and what is its original rate per metre ? (Marks
4)
Q20) A sphere of diameter 6
cm is dropped in a right circular cylindrical vessel partly filled with
water. The diameter of the cylindrical vessel is 12 cm. If the sphere is
completely submerged in water, by how much will the level of water rise in
the cylindrical vessel ? (Marks 4)
|
