|
Maths Class X 2000(CBSE)
You are on Set no 1 Qno. 21 to 30
Q21) Show that 2sec2  - sec4 - sec4  - 2cosec2 - 2cosec2  +
cosec4 +
cosec4  = cot4 = cot4  - tan4 - tan4  (Marks 4) (Marks 4)
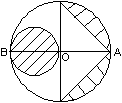
Q22) In fig. AB is a diameter of circle with centre O, OA = 7 cm.
Find the area of the shaded region ( use 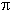 = 22/7). (Marks 4) = 22/7). (Marks 4)
Q23)
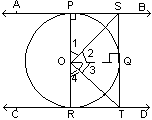
In fig. AB and CD
are two parallel tangents to a circle with centre O. ST is tangent segment
between two parallel tangents touching the circle at Q. Show that  SOT =
90o. (Marks 4) SOT =
90o. (Marks 4)
Q24)
Construct a quad.
ABCD in which AB = 2.5 cm BC = 3.5 cm, AC = 4.2 cm, CD = 3.5 cm and AD =
2.5 cm. Construct another quad. AB'C'D' with diagonal AC' = 6.3 cm such
that it is similar to quad ABCD. (Marks 4)
Q25) Find the cost of living index number for the year 1995 assuming
1990 as the base year. (Marks 4)
| Commodity |
Quantity
(kg) |
Rate per kg (in
Rs.) |
| 1990 |
1995 |
| A |
10 |
7 |
10 |
| B |
15 |
12 |
20 |
| C |
8 |
25 |
25 |
| D |
25 |
12 |
20 |
| E |
5 |
50 |
60 |
Section - C
Q26) Solve for x : 9x + 2 - 6 x 3x + 1 + 1 =
0 (Marks 6)
Q27)
If a radius of the circular and of a conical bucket, which is
45 cm high are 28 cm, 7 cm, find the capacity of the bucket.
(Marks 6)
Q28)
Prove that ratio
of area of two similar triangles is equal to the ratio of squares of
corresponding sides. Using the above do the following :-
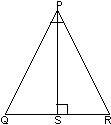
In fig  ABC and ABC and  PQR are
isosceles in which A = P PQR are
isosceles in which A = P
If ar( ABC)/ar( ABC)/ar( PQR) = 9/16, find AD/PS (Marks
6) PQR) = 9/16, find AD/PS (Marks
6)
Q29)
Prove that sum of either pair of opposite angles of a cyclic
quadrilateral is 180o. Using the above solve the
following:

In fig POQ is a diameter and PQRS is a cyclic quadrilateral.
If  PSR =
150o, find PSR =
150o, find  RPQ. (Marks 6) RPQ. (Marks 6)
Q30) A man on the roof
of a home, which is 10 m high, observes the angle of elevation of the top
of a building as 42o and angle of depression of the base of the
building as 40o. Find the height of the building and its
distance from the home. (Marks 6)
|
